Introduction
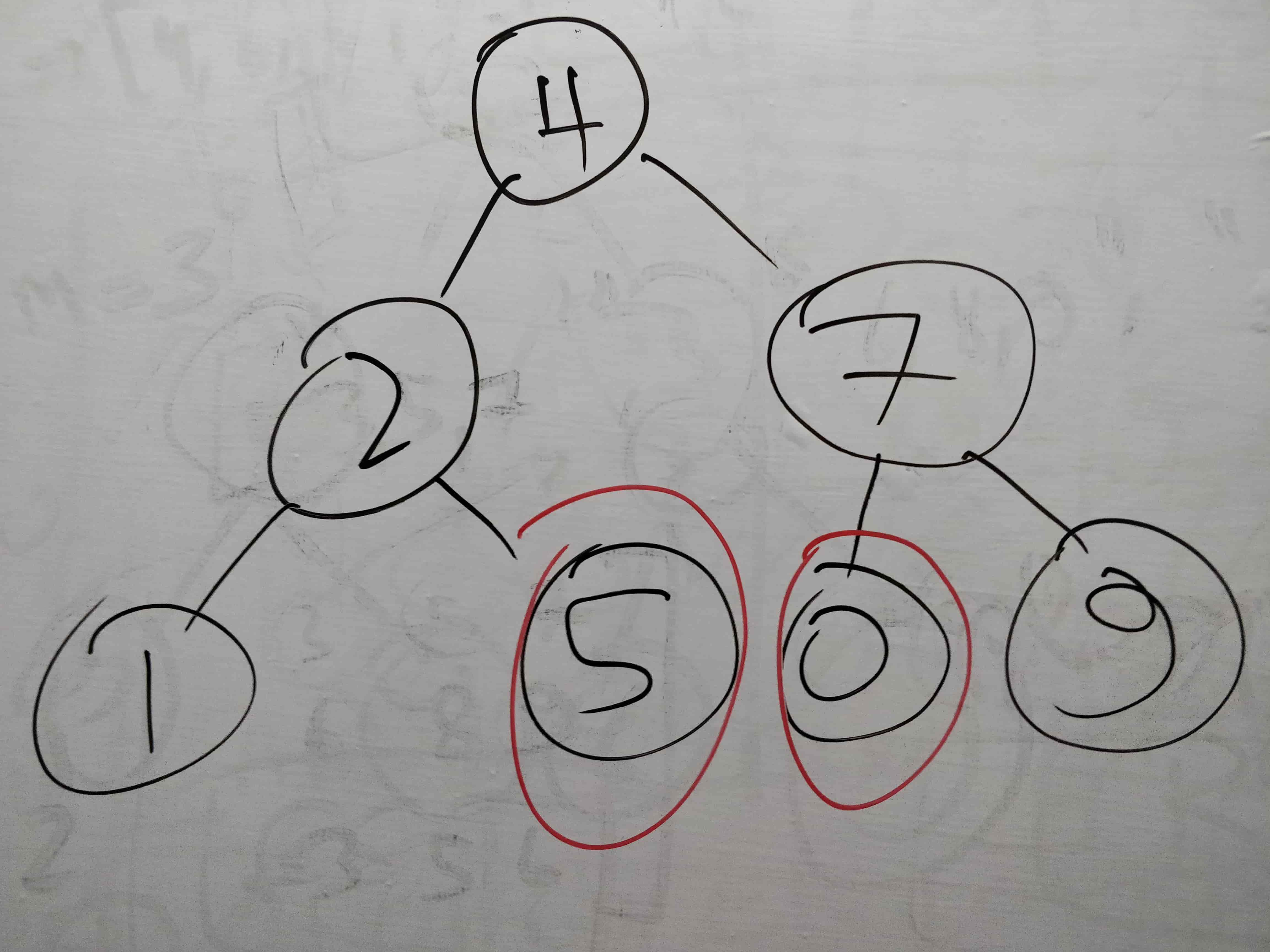
Given a binary tree, determine if it is a valid binary search tree (BST). Let's discuss more about some of the inputs.
2
/ \
1 3
Output: true
5
/ \
1 4
/ \
3 6
Output: false
Explanation: The root node's value is 5 but its right child's value is 4.Approach
We consider the approach to keep track of minimum and maximum values allowed for any node.
The program performs the in-order traversal and in each recursive call, minimum and maximum range is passed. The range checks whether the current node’s value is within the given range.
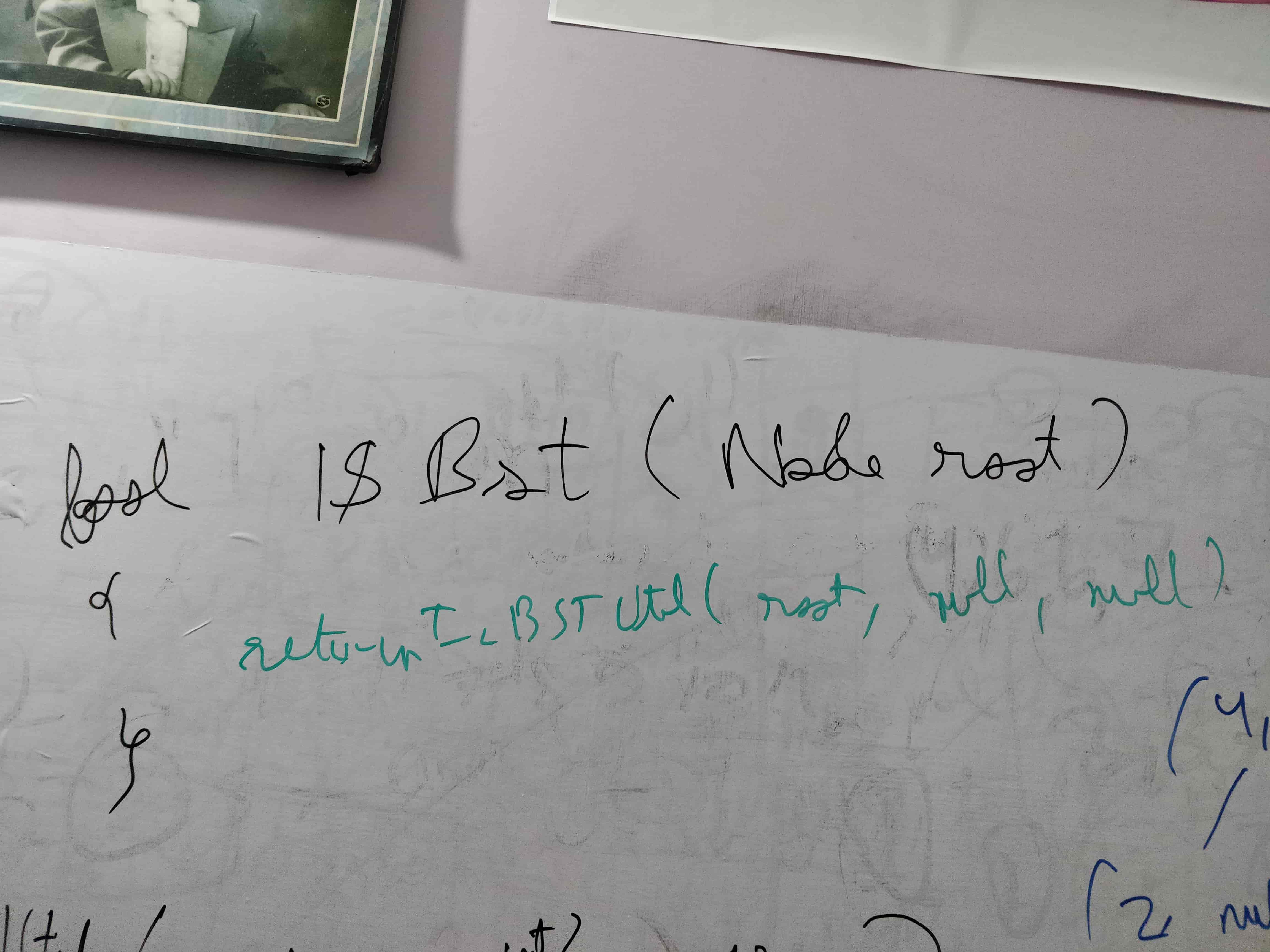
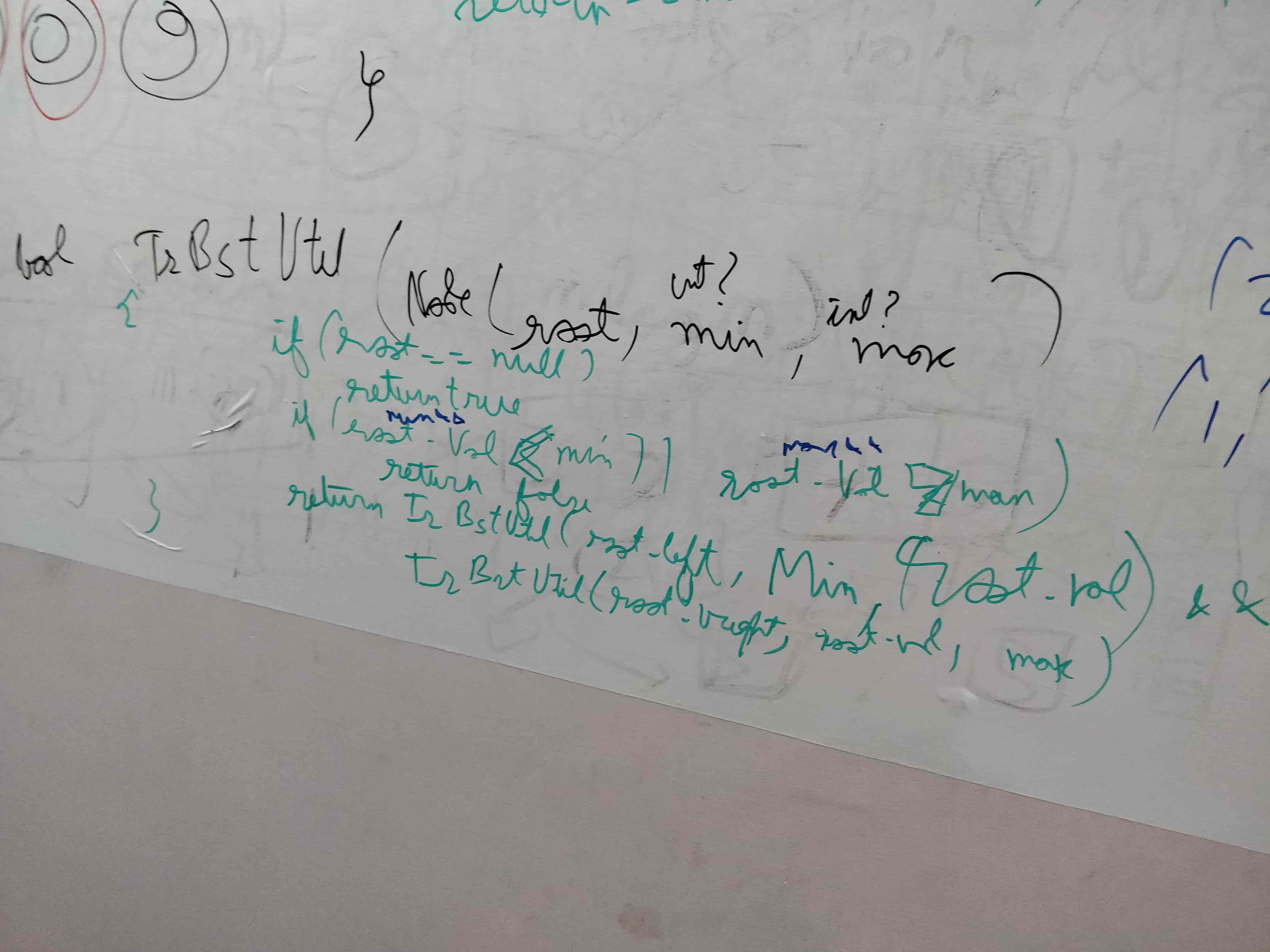
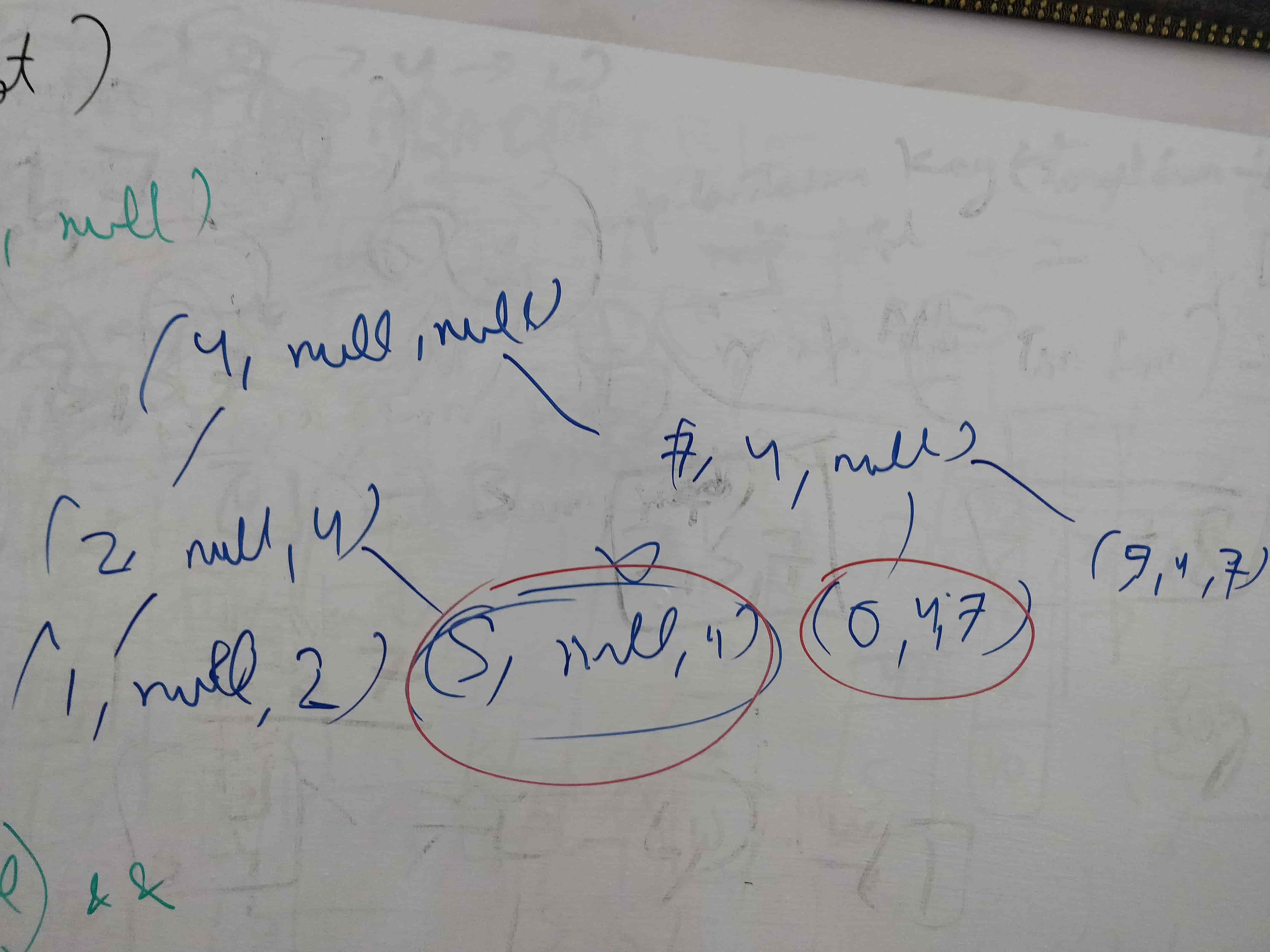
/**
* Definition for a binary tree node.
* public class TreeNode {
* public int val;
* public TreeNode left;
* public TreeNode right;
* public TreeNode(int val=0, TreeNode left=null, TreeNode right=null) {
* this.val = val;
* this.left = left;
* this.right = right;
* }
* }
*/
public class Solution {
public bool IsValidBST(TreeNode root) {
return IsValidBstUtil(root, null, null);
}
private bool IsValidBstUtil(TreeNode node, int? min, int? max) {
if (node == null) {
return true;
}
if ((min != null && node.val <= min.Value) || (max != null && node.val >= max.Value)) {
return false;
}
return IsValidBstUtil(node.left, min, node.val) &&
IsValidBstUtil(node.right, node.val, max);
}
}
